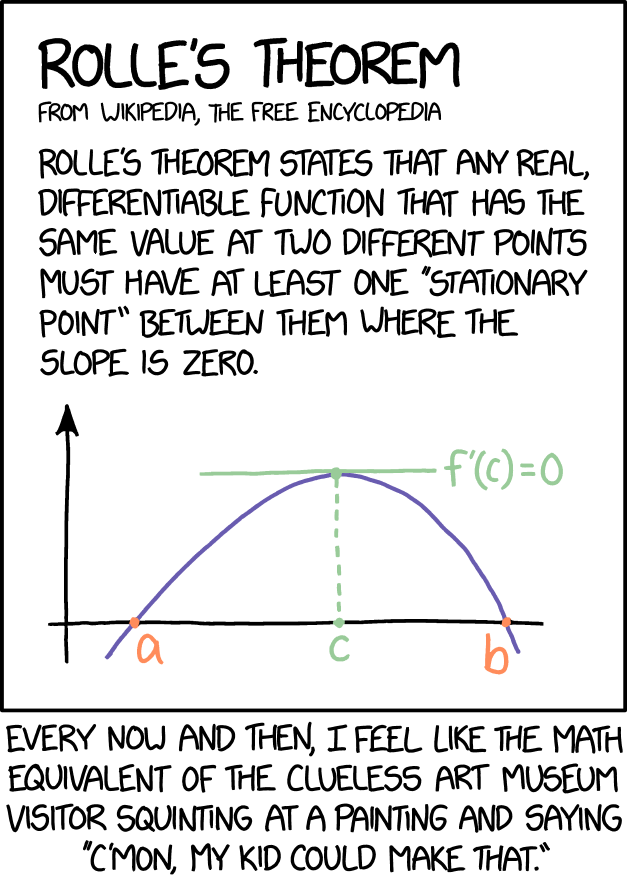
yea this is one of those theorems but history is studded with "the proof is obvious" lemmas that has taken down entire sets of theorems (and entire PhD theses)
this post was submitted on 20 Oct 2024
7 points (100.0% liked)
Math Memes
1998 readers
367 users here now
Memes related to mathematics.
Rules:
1: Memes must be related to mathematics in some way.
2: No bigotry of any kind.
founded 2 years ago
MODERATORS
This guy would not be happy to learn about the 1+1=2 proof
One part of the 360 page proof in Principia Mathematica:
It's not a 360 page proof, it just appears that many pages into the book. That's the whole proof.
Weak-ass proof. You could fit this into a margin.
Upvoting because I trust you it's funny, not because I understand.
It's a reference to Fermat's Last Theorem.
Tl;dr is that a legendary mathematician wrote in a margin of a book that he's got a proof of a particular proposition, but that the proof is too long to fit into said margin. That was around the year 1637. A proof was finally found in 1994.
I thought it must be something like that, I expected it to be more specific though :)
late to the party, but that's actually one of the heartwarming and ridiculous at the same time stories of mathematics
Principia mathematica should not be used as source book for any actual mathematics because it’s an outdated and flawed attempt at formalising mathematics.
Axiomatic set theory provides a better framework for elementary problems such as proving 1+1=2.
Yeah, the four color problem becomes obvious to the brain if you try to place five territories on a plane (or a sphere) that are all adjacent to each other. (To require four colors, one of the territories has to be surrounded by the others)
But this does not make for a mathematical proof. We have quite a few instances where this is frustratingly the case.
Then again, I thought 1+1=2 is axiomatic (2 being the defined by having a count of one and then another one) So I don't understand why Bertrand Russel had to spend 86 pages proving it from baser fundamentals.
Then again, I thought 1+1=2 is axiomatic (2 being the defined by having a count of one and then another one) So I don't understand why Bertrand Russel had to spend 86 pages proving it from baser fundamentals.
Well, he was trying to derive essentially all of contemporary mathematics from an extremely minimal set of axioms and formalisms. The purpose wasn't really to just prove 1+1=2; that was just something that happened along the way. The goal was to create a consistent foundation for mathematics from which every true statement could be proven.
Of course, then Kurt Gödel came along and threw all of Russell's work in the trash.
Saying it was all thrown in the trash feels a bit glib to me. It was a colossal and important endeavour -- all Gödel proved was that it wouldn't help solve the problem it was designed to solve. As an exemplar of the theoretical power one can form from a limited set of axiomatic constructions and the methodologies one would use it was phenomenal. In many ways I admire the philosophical hardball played by constructivists, and I would never count Russell amongst their number, but the work did preemptively field what would otherwise have been aseries of complaints that would've been a massive pain in the arse
You only needed to choose 2 points and prove that they can't be connected by a continuous line. Half of your obviousness rant
prove it then.
It's fucking obvious!
Seriously, I once had to prove that mulplying a value by a number between 0 and 1 decreased it's original value, i.e. effectively defining the unary, which should be an axiom.
So you need to proof x•c < x for 0<=c<1?
Isn't that just:
xc < x | ÷x
c < x/x (for x=/=0)
c < 1 q.e.d.
What am I missing?
My math teacher would be angry because you started from the conclusion and derived the premise, rather than the other way around. Note also that you assumed that division is defined. That may not have been the case in the original problem.
Your math teacher is weird. But you can just turn it around:
c < 1
c < x/x | •x
xc < x q.e.d.
This also shows, that c≥0 is not actually a requirement, but x>0 is
I guess if your math teacher is completely insufferable, you need to add the definitions of the arithmetic operations but at that point you should also need to introduce Latin letters and Arabic numerals.
Mathematicians like to have as little axioms as possible because any axiom is essentially an assumption that can be wrong.
Also proving elementary results like your example with as little tools as possible is a great exercise to learn mathematical deduction and to understand the relation between certain elementary mathematical properties.
A lot of things seem obvious until someone questions your assumptions. Are these closed forms on the Euclidean plane? Are we using Cartesian coordinates? Can I use the 3rd dimension? Can I use 27 dimensions? Can I (ab)use infinities? Is the embedded space well defined, and can I poke a hole in the embedded space?
What if the parts don't self-intersect, but they're so close that when printed as physical parts the materials fuse so that for practical purposes they do intersect because this isn't just an abstract problem but one with real-world tolerances and consequences?
Yes, the paradox of Gabriel's Horn presumes that a volume of paint translates to an area of paint (and that paint when used is infinitely flat). Often mathematics and physics make strange bedfellows.